| Type of snow or ice | (kg/m3) | |
| New snow | 50-70 | |
| Damp new snow | 100-200 | |
| Settled snow | 200-300 | |
| Depth hoar | 100-300 | |
| Wind packed snow | 350-400 | |
| Firn (granular) | 400-830 | |
| Very wet | 700-800 | |
| Glacier ice | 830-917 |
The Weight of Snow on a Rectangular or Square Area calculator computes approximate weight of snow on an rectangular area (e.g. roof) based on the dimensions of the rectangle covered (length times width), the depth of the snow, and the type of snow (based on standard snow types).
INSTRUCTIONS: Choose your preferred units and enter the following:
- (L) This is the length of the rectangular area.
- (W) This is the width of the rectangular area.
- (D) This is the depth of the snow.
- (ST) This it the type of snow (see list)
Weight of Snow: The weight of the snow is returned in kilograms. However, this can be automatically converted to other weight units (e.g. pounds or tons) via the pull-down menu.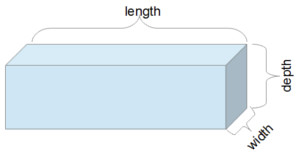
Related Calculators:
- To compute the weight of snow on an area, CLICK HERE.
- To compute the weight of snow on a polygon shaped area, CLICK HERE.
- To compute the weight of snow on a roof, CLICK HERE.
- To compute the amount of water in snow laying on land, CLICK HERE.
Notes
Snow Water Equivalent (SWE) is the product of snow depth and snow density. It can be presented in units of either kg/m2 or m:
SWE (`(kg)/(m^2)`) = snow depth (m) x snow density (`(kg)/(m^3)`)
SWE (m) = snow depth (m) x snow density (`(kg)/(m^3)`) / water density (`(kg)/(m^3)`)
You can calculate snow depth from SWE if you know the density of the snow. Od course, density of snow can range anywhere from 5% when ambient air temperature is 14 F, and can range up to 20% if the temperature is 32 F.
The snow density will increase after the snowfall due to gravitational settling, packing, wind effects, melting and refreezing.
| Type of snow or ice | densities (kg/m³) |
| New snow (immediately after falling in calm) | 50-70 |
| Damp new snow | 100-200 |
| Settled snow | 200-300 |
| Depth hoar | 100-300 |
| Wind packed snow | 350-400 |
| Firn (granular snow) | 400-830 |
| Very wet snow and firn | 700-800 |
| Glacier ice | 830-917 |
The equation will use a median value for the ranges in the density value column.